How to Use the Angle-Bisector Theorem

The Angle-Bisector theorem states that if a ray bisects an angle of a triangle, then it divides the opposite side into segments that are proportional to the other two sides. The following figure illustrates this.

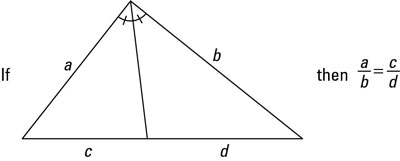
The Angle-Bisector theorem involves a proportion — like with similar triangles. But note that you never get similar triangles when you bisect an angle of a triangle (unless you bisect the vertex angle of an isosceles triangle, in which case the angle bisector divides the triangle into two congruent triangles).

Don’t forget the Angle-Bisector Theorem. (For some reason, students often do forget this theorem.) So whenever you see a triangle with one of its angles bisected, consider using the theorem.

How about an angle-bisector problem? Why? Oh, just BCUZ.

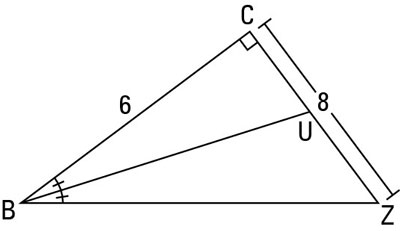
Given: Diagram as shown

Find: 1.) BZ, CU, UZ, and BU and 2.) The area of triangle BCU and triangle BUZ

1. Find BZ, CU, UZ, and BU.

   
   
   

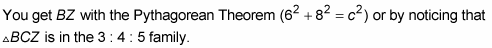
   It’s a 6-8-10 triangle, so BZ is 10.

   
   

   Next, set CU equal to x. UZ then becomes 8 – x. Set up the angle-bisector proportion and solve for x:

   
   
   

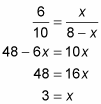
   So CU is 3 and UZ is 5.

   
   

   The Pythagorean Theorem then gives you BU:

   
   
   



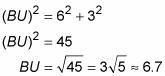
2. Calculate the area of triangle BCU and triangle BUZ.

   
   

   Both triangles have a height of 6 (when you use segment CU and segment UZ as their bases), so just use the triangle area formula:

   
   
   

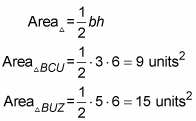
Note that the ratio of the areas of these triangles, 9 : 15 (which reduces to 3 : 5), is equal to the ratio of the triangles’ bases, 3 : 5. This equality holds whenever a triangle is divided into two triangles with a segment from one of its vertices to the opposite side (whether or not this segment cuts the vertex angle exactly in half).

dummies

Source:http://www.dummies.com/how-to/content/how-to-use-the-anglebisector-theorem.html