How to Calculate the Area of a Kite

You calculate the area of a kite by using the lengths of its diagonals. Here’s an example: what’s the area of kite KITE in the following figure?

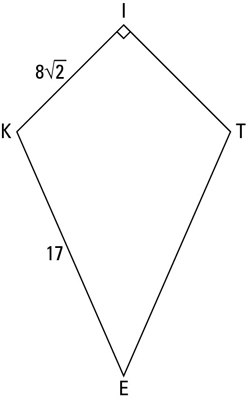
For kite area problems (and sometimes other quadrilateral problems), the diagonals are almost always necessary for the solution (because they form right triangles). So if the given diagram doesn’t show the diagonals, you should draw them in yourself.

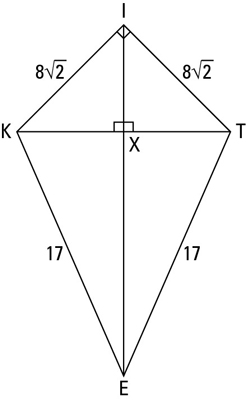
Draw in segment KT and segment IE as shown in the above figure.

Triangle KIX is another 45°- 45°- 90° triangle (segment IE, the kite’s main diagonal, bisects opposite angles KIT and KET, and half of angle KIT is 45°); therefore, IX, like KX, is 8.

You’ve got another right triangle, KXE, with a side of 8 and a hypotenuse of 17. Hopefully that rings a bell! You’re looking at an 8-15-17 triangle, so without any work, you see that XE is 15. (No bells? No worries. You can get XE with the Pythagorean Theorem instead.)

You determined the length of the other diagonal above, so you’ve got what you need for the kite area formula.

Plug these numbers into the formula for your final answer:

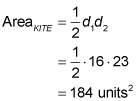
dummies

Source:http://www.dummies.com/how-to/content/how-to-calculate-the-area-of-a-kite.html