One of the nice qualities of right triangles is that you can use trigonometry to find the length of one side if you know the lengths of the other two sides. You don’t have this luxury with just any triangle, so count your blessings now.
>
 >
>
Solving for the missing sides.
The Pythagorean theorem states that a2 + b2 = c2 in a right triangle where c is the longest side. You can use this equation to figure out the unknown length of one side. The preceding figure shows two right triangles that are each missing one side’s measure.
In the left triangle in the preceding figure, the measure of the hypotenuse is missing. Use the Pythagorean theorem to solve for the missing length.
Replace the variables in the theorem with the values of the known sides.
482 + 142 = c2
Square the measures and add them together.

Find the square root of each side of the equation.
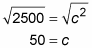
The length of the missing side, c, which is the hypotenuse, is 50.
Technically, taking the square root of each side of an equation gives you both positive and negative answers. But a negative length doesn’t make sense, so you just use the positive answer.
The triangle on the right in the preceding figure is missing the bottom length, but you do have the length of the hypotenuse. It doesn’t matter whether you call the missing length a or b.
Replace the variables in the theorem with the values of the known sides.
332 + b2 = 1832
Square the measures, and subtract 1089 from each side.

Find the square root of each side of the equation.
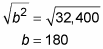
The length of the missing side is 180 units. That’s not much shorter than the hypotenuse, but it still shows that the hypotenuse has the longest measure.
>
dummies
Source:http://www.dummies.com/how-to/content/how-to-solve-for-a-missing-right-triangle-length.html
No comments:
Post a Comment